
Civil Engineering Software, Training and Consultancy

Civil Engineering Software, Training and Consultancy
Finding out the effective area and the required size of foundation meeting bearing capacity and other serviceability requirements is time consuming and iterative task. Above that, if we want to calculate the required
size of foundation common for multiple number of supports or load combinations in STAAD is again a tedious job.
Providing foundation sizes for maximum forces may lead to provision of bigger size of foundation.
This automation tool enables user to arrive at the most optimized size
of footing meeting all the recommendations set by user in no time.
This tool is developed taking into consideration all the possible
requirements of civil engineer who wants to analyze the footing
for a group of nodes against a set of project specific load combinations.
This tool gives user freedom of choosing the shape of footing whether
he wants to go ahead with square footing or rectangular footing.
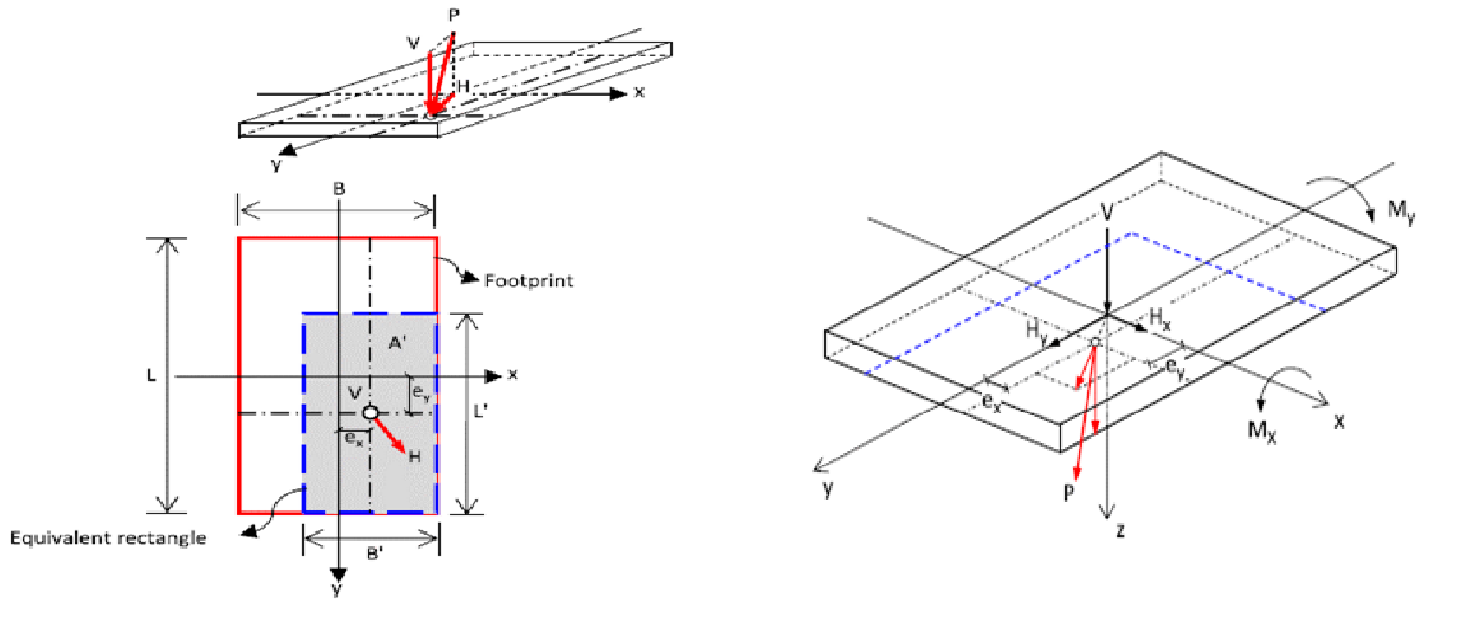
The effective width hypothesis was proposed by Meyerhof (1953)
for strip foundations to consider the detachment of the foundation
due to overturning moment resulting from eccentricity of vertical
load. The concept of an effective area has since been developed
for other foundation geometries (Hansen 1961; Taiebat and Carter
2002). Figure mentioned below depicts a rectangular foundation subjected to a point
load P. The eccentric-inclined load P is decomposed into a vertical
component V normal to the base and a horizontal component H,
intersecting the center of an equivalent fictitious rectangle for
which the bearing capacity is calculated. The equivalent rectangle
is determined such that the geometrical center of the rectangle
coincides with the point of load application, and the rectangle follows
as closely as possible the nearest contour of the actual bearing area
(Hansen 1970). The load inclination is included by further reducing
the bearing capacity of the rectangle. Then, the load-carrying
capacity of a rectangular foundation is defined as the pure vertical
capacity of the equivalent rectangle.
where A' = effective area of the foundation, which is equal to B'L';
and B' and L' = minimum and maximum effective lateral foundation
dimensions depending on the load eccentricity, respectively
B' = Min(B - 2ex; L - 2ey); L' = Max(B - 2ex; L - 2ey)
where ex and ey = eccentricities of the vertical load along the short
(B) and long (L) side of the rectangular foundation, respectively, with
ex = -My/V; ey = Mx/V
Download 15 Days Trail version of the product, register and activate on your PC today. (see, how to register and activate the Product)
No credit card details required to be submitted for Trial Version. After 15 days of trial period, you can choose the plan suitable for you anytime and activate it again.
*Updates related to compatibility issue with system version.
Read License Agreement and License Agreement FAQs